Understanding Amortisation:

Amortisation is an essential financial concept that is used to manage loans, mortgages, and other debts. When you hear the term, it generally means the act of repaying a loan over a specific period of time, through regular payments that include both the original amount borrowed and the interest accrued.

This article will explore the concept of Amortisation in detail, including its definition, formula, types, and examples.

Definition:

Amortisation refers to the accounting process of allocating the cost of a long-term asset over its useful life. This is done to reflect the gradual consumption of the asset's value over time and to accurately match expenses with revenue generated by the asset. In other words, it is a method used to reduce the value of a loan or an asset over time through regular payments. The payments made towards the principal and interest of the loan are usually equal and paid at regular intervals, such as monthly or quarterly.

Formula:

The formula for calculating the Amortisation of a loan is as follows: A = P * r * (1 + r) ^ n / ((1 + r) ^ n - 1)

Where: A = the regular payment or Amortisation amount

P = the loan principal amount

i = the interest rate per period

n = the total number of payment periods

Types of Amortisation:

There are two main types of Amortisation: straight-line and declining balance.

• Straight-line Amortisation: This method of Amortisation involves equal payments over the life of the loan, with each payment consisting of both principal and interest. The calculation of interest on a loan involves multiplying the remaining balance of the loan by the applicable interest rate. On the other hand, the principal amount can be determined by subtracting the interest amount from the total payment.
• Declining balance Amortisation: This method of Amortisation involves a higher payment in the beginning, with the payment amount gradually decreasing over time. The reason for this is that the interest on the loan is calculated according to the remaining balance, which diminishes as the borrower makes payments towards the principal. As a result, the principal amount paid off with each payment gradually increases.

Examples: Let's consider an example to illustrate the concept of Amortisation: Suppose you took out a loan of $10,000 with an interest rate of 5% per year, to be repaid over a period of 5 years. Using the formula above, we can calculate the monthly payment or Amortisation amount as follows:

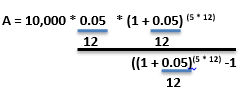
= $188.71

This means that you would have to pay $188.71 every month for 5 years to fully repay the loan. Payments towards the loan would include both the principal amount and interest. The interest component gradually reduces over time as the outstanding loan balance is paid off.

Conclusion: In conclusion, Amortisation is a critical financial concept that is used to manage loans, mortgages, and other debts. The process of allocating the cost of an asset or loan over its useful life by making periodic payments that include both principal and interest is commonly known as Amortisation. There are two main types of Amortisation: straight-line and declining balance, each with its unique features and benefits. Having a grasp of how Amortisation works is vital for individuals who aim to effectively handle their finances and arrive at well-informed financial choices.

Customer Notice:

Nextgen Global Services Pty Ltd trading as Kapitales Research (ABN 89 652 632 561) is a Corporate Authorised Representative (CAR No. 1293674) of Enva Australia Pty Ltd (AFSL 424494). The information contained in this website is general information only. Any advice is general advice only. No consideration has been given or will be given to the individual investment objectives, financial situation or needs of any particular person. The decision to invest or trade and the method selected is a personal decision and involves an inherent level of risk, and you must undertake your own investigations and obtain your own advice regarding the suitability of this product for your circumstances. Please be aware that all trading activity is subject to both profit & loss and may not be suitable for you. The past performance of this product is not and should not be taken as an indication of future performance.

Kapitales Research, Level 13, Suite 1A, 465 Victoria Ave, Chatswood, NSW 2067, Australia | 1800 005 780 | info@kapitales.com